Holy shit, I need to do this.

So today I googled "cumulative graph" and ended up rethinking my philosophy and practice of teaching. Doesn't happen every day, to say the least.

Here's how it happened: "cumulative graph" lead to this site, which introduced me not only to what seems to be an outstanding researcher and communicator, Malcolm Swan, but also to a whole set of awesome activities for teaching statistics, algebra, and other things.

Malcolm Swan thus really caught my interest, and google led me to his summary of effective teaching strategies which is extremely user friendly for teachers. What really made me cringe and laugh and decide to shake things up was this collection of powerpoint slides, and especially his comparison of "guided discovery" (what I do a lot of) and "conflict and discussion" (which I mostly do by accident).

To compare the two methods, Swan shows us a problem from from Brekke, 1986, but I think the correct  reference is: "Bell, A., Brekke, G., & Swan, M. (1987). Misconceptions, conflict and discussion in the teaching of graphical interpretation." (If someone can find the full article/book excerpt I'd be most grateful, because it's not available through my university library database.)

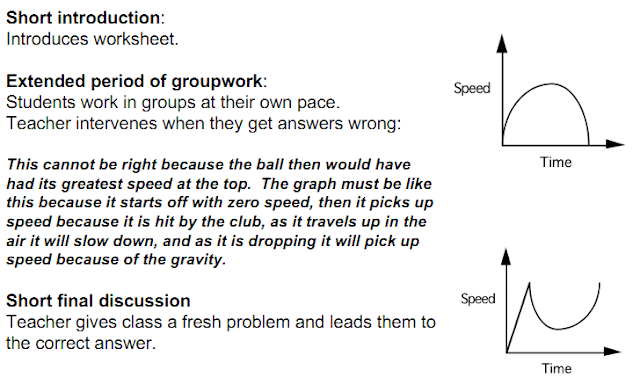
The Guided Discovery approach: 

Ouch. I do that. I thought this was the way to do it, you know, guided discovery is the shit, right?

But when someone puts it like this, I don't want to do it no more. 

By contrast, the Conflict and Discussion approach involves

1. Individual work
2. Discussion in small group (opportunity for conflict when group members have different suggestions)
3. Writing about what happens in the problem
4. Drawing a graph
5. Interpreting the graph back into words  (opportunity for conflict when words don't match the writing in step 3)
6. Discussing the solution with the whole class (opportunity for conflict when classmates have other suggestions)
7. Final (long) discussion about what common errors people make, and why. 

Each time there is conflict, students restart from step 1. 

Results-wise, Swan cites two studies that find that student concept attainment (as measured by pre- and post-tests) is greatly increased in the Conflict and Discussion method. Astoundingly, students retain their understanding even months after the lesson. I think that's what convinced me that give this a serious try. 

OK, so how? Well, conflict seems to me to come from several sources: 

1. Students self-check and find that their solution doesn't work or make sense
2. Comparison with other students reveal conflicting solutions 
3. Students are shown examples of something that they have a hard time fitting into prior concept.

Building these conflicts seems to require the teacher to above all lay off giving the answer, and instead focus on challenging the students with prompts such as "check if it makes sense" and "compare with that guy" or "well how does .... fit with what you're saying?". Actually, I think another way to create conflict is telling the student that his solution makes no sense or is incorrect (even if it's perfectly correct and makes perfect sense) and then just walking away. I'm not sure that will breed a healthy type of frustration in all students, though. 

My main problem, as usual, is that discussions take time, and time I don't have. Swan argues that according to research results, it's more effective to focus on just a few problems in depth than many similar-but-slightly-different questions superficially. I'm going to try this, so let's hope he's right.